The co-evolution of multiply-informed dispersal: information transfer across landscapes from neighbors and immigrants

Introduction

Dispersal is a key component of many ecological and evolutionary processes ranging from population dynamics to local adaptation and has been the focus of extensive empirical and theoretical investigation (Clobert et al., 2001; Ronce, 2007; Nathan et al., 2008; Clobert et al., 2012). The impact of dispersal on population dynamics, movement across the landscape, and local adaptation makes it a critical element of understanding how populations are affected by landscape fragmentation and global warming (Chaine & Clobert, 2012). Dispersal has largely been treated as a population level character even though dispersal decisions are fundamentally an individual behavior that should benefit from knowledge of the landscape. Recent empirical evidence suggests that information use in making dispersal decisions and navigating the landscape plays an important role in patterns of dispersal (Bowler & Benton, 2005; Clobert et al., 2009; Schmidt, Dall & Van Gils, 2010). Information use would cause a shift in how we view dispersal. Exchanges among populations would no longer represent a random subset of genotypes and might affect local adaptation patterns (Blanchet, Clobert & Danchin, 2010). Dispersers might not spread randomly across the landscape and some populations might receive more or fewer immigrants due to dispersal costs (Bonte et al., 2012). In applied work, if we want to encourage dispersal, we would need to make sure that the key information sources are available or even manipulate information to get the desired level of dispersal (Blanchet, Clobert & Danchin, 2010; Chaine & Clobert, 2012). Yet our fundamental understanding of informed dispersal remains limited (Clobert et al., 2009).

The use of information in dispersal decisions has received attention through a limited range of possibilities despite potentially important effects on fitness (Ims & Hjermann, 2001; Ronce et al., 2001; Bowler & Benton, 2005; Clobert et al., 2009; Schmidt, Dall & Van Gils, 2010). Classical ecological (metapopulation) and evolutionary (gene-flow) theory assumes constant dispersal rates with random movement and no information use (Hanski & Gaggiotti, 2004). At the other extreme, ideal free settlement models assume perfect knowledge of the entire landscape which influences dispersal (Holt & Barfield, 2001). Both approaches are analytically tractable, but biologically unrealistic since organisms often use some information (Greene, 1987; Danchin et al., 2004; Dall et al., 2005; Avarguès-Weber, Dawson & Chittka, 2013) but rarely have perfect information. Significant progress in understanding dispersal itself will require specific attention to biologically plausible mechanisms for gathering information (Schmidt, Dall & Van Gils, 2010).

Recent models have investigated how information on local population density affects dispersal (Travis, Murrell & Dytham, 1999; Cadet et al., 2003; Ronce, 2007; Enfjäll & Leimar, 2009; Hovestadt, Kubisch & Poethke, 2010; Bocedi, Heinonen & Travis, 2012), but it is becoming increasingly clear that organisms use a variety of information sources (Ronce et al., 2001; Danchin et al., 2004; Bonnie & Earley, 2007; Clobert et al., 2009; Schmidt, Dall & Van Gils, 2010) that might inform them about the presence or content of other populations in the landscape without direct measurement. We call these forms of information that do not result from direct sampling of the environment “indirect information” (Doligez, Danchin & Clobert, 2002; Danchin et al., 2004; Blanchet, Clobert & Danchin, 2010). For example, tourists in Paris are easily identified by the fact that they are using maps (unlike Parisians) and this might suggest to Parisians that there is indeed a habitable world outside of Paris. These more “indirect” sources of information derived from the observation of conspecifics are more controversial because they less accurately predict fitness in any given patch (Schmidt, Dall & Van Gils, 2010). However, indirect information carries a distinct advantage of providing some information about other patches without requiring costly exploration of other sites.

A few recent empirical examples in birds, lizards, and other organisms now suggest that indirect social information is accessible and used by individuals in making dispersal decisions (Doligez, Danchin & Clobert, 2002; Cote & Clobert, 2007a; Chaine et al., 2010; De Meester & Bonte, 2010). For example, in the common lizard (Zootoca vivipara), juveniles use a number of direct and indirect sources of information to make dispersal decisions (Clobert, Massot & Le Galliard, 2012). Juveniles gain direct information by sampling the density of their patch (Le Galliard, Ferriere & Clobert, 2003) and regarding kin competition (especially mother-offspring competition; Léna et al., 1998; De Fraipont et al., 2000). However, juveniles also gain indirect information based on the arrival of new immigrants (Cote & Clobert, 2007b; Cote, Boudsocq & Clobert, 2008; Cote & Clobert, 2012) and the failure of emigrants to find new populations (Cote & Clobert, 2007a). Likewise, some spider species, use both direct sampling of information on density, habitat quality, and wind direction (Bonte et al., 2003a; Bonte et al., 2003b; Bonte, Bossuyt & Lens, 2007; Bonte, Van Belle & Maelfait, 2007; De Meester & Bonte, 2010) as well as indirect information such as the number of other individuals dispersing (De Meester & Bonte, 2010). Yet it remains unclear how prevalent use of indirect information in dispersal might be across species. Widespread use of indirect information would dramatically alter our understanding of dispersal and would have consequences for both fundamental work in ecology and evolution as well as applied conservation.

Using a theoretical model, we show that simple rules for the use of indirect social information in dispersal decisions can evolve under a broad range of conditions and therefore might be quite common in nature. We investigated the evolution of information use prior to dispersal using a simple metapopulation model in which we allowed information use in dispersal to evolve. We were primarily interested in whether the use of indirect information provided by immigrants could evolve, and if so, could it evolve in competition with direct information about local density.

The model

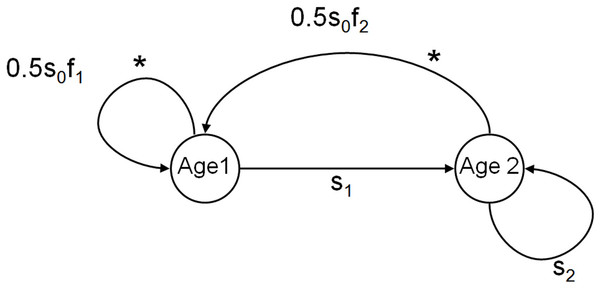
We constructed an individual-based model of informed dispersal behavior, based on information about the local density and/or the number of immigrants, while simplifying the landscape and genetic features of the system. This individual-based model follows a female-based life cycle with two age-classes (individuals in the population are juveniles from birth until age 1, subadults from age 1 to 2, and adults after age 2 and age-specific survival and fecundity (Caswell, 2001, and see Fig. 1). Only juveniles dispersed and this dispersal depended on baseline uninformed dispersal that alleviates kin competition (U) and informed dispersal (D and I) as described below. Our basic model used a “fast” life history roughly equivalent to a small lizard or passerine life cycle (survival: s₀ = 0.2, s₁ = 0.35, s₂ = 0.5; fecundity: f₁ = 7, f₂ = 7, see Schoener et al., 2003; Legendre et al., 2008). In each patch, discrete time structured population dynamics were modeled. Juveniles were given the opportunity to disperse to other patches prior to the subsequent reproductive episode if they survived their first year. All patches were equally connected (leading to lower kin competition) and population size was limited at reproduction by the maximum patch carrying capacity which was the same for all patches (K = 100). This configuration leads to very stable populations with low levels of demographic stochasticity, lower kin competition, and very small benefits of dispersal (populations are all similarly near K) essentially creating a conservative scenario for the evolution of informed dispersal. Subsequent simulations introduced increased stochasticity to explore the benefits of information use under other scenarios (see SOM).

Results

Evolution of information use: single source of information

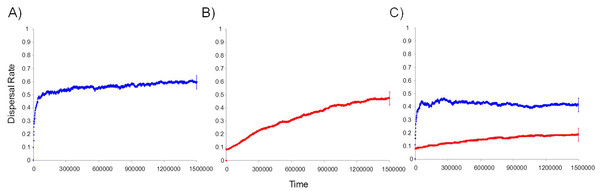
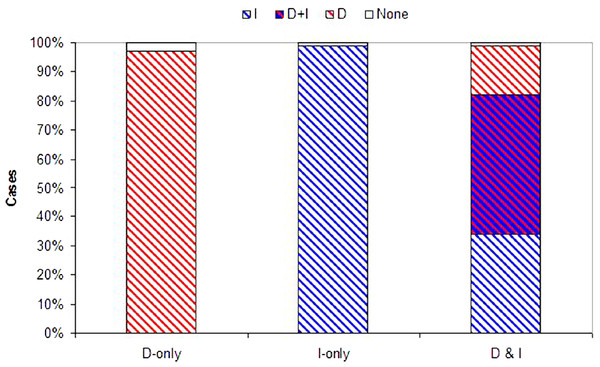
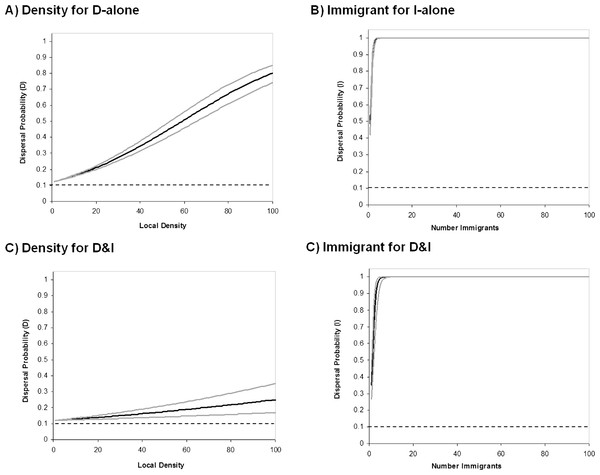
We found that informed dispersal could evolve and drive dispersal behavior and metapopulation dynamics under a broad range of contexts. Consistent with other models (Travis, Murrell & Dytham, 1999; Ronce, 2007), we found that density dependent dispersal evolves when it is the only source of information (Figs. 2A, 3). Here we show that the arrival of immigrants also provides useful information that can drive dispersal behavior (Figs. 2B, 3). Indeed, information-dependent dispersal coefficients (D and I) were significantly biased towards positive values in contrast to expectations from drift which should lead to an equal probability of positive and negative values (Sign test: D-only: 97/100 positive trials, P < 0.0001; I-only: 99/100 positive trials, P < 0.0001). Both density and immigrant dependent dispersal evolved even when each was in competition with uninformed dispersal (fixed d_U = 10% and when U was allowed to evolve; see SOM and Figs. S9, S10a) and lead to increased dispersal from that source of information (Figs. 2A, B) despite a highly stable and homogenous landscape. Dispersal reaction norms due to information use illustrate this nicely: local density and immigrant number influence dispersal (Fig. 4A, B respectively) at equilibrium compared to a flat, fixed dispersal rate of uninformed dispersal. Density-dependent dispersal shows a steady increase in dispersal as local density rises (Fig. 4A). In contrast, immigrant-dependent dispersal shows a rapid increase in dispersal with the first few immigrants and then quickly asymptotes at high levels of dispersal (Fig. 4B).

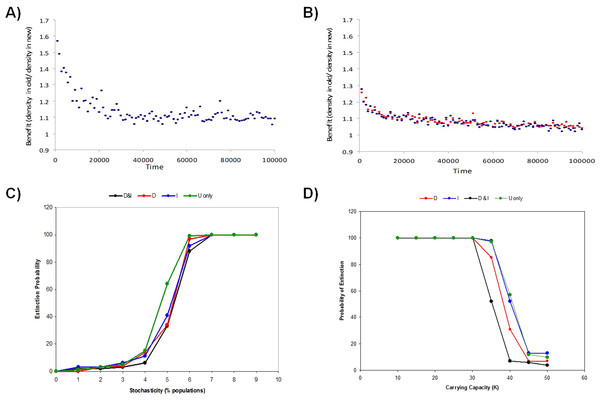
For informed dispersal to evolve there must be some benefit to these strategies. Individuals benefit from dispersal when they find a new population with a lower density given that fitness is density-dependent. We compared the density of the new destination patch and an individual’s original patch right before reproduction to estimate the benefit of dispersal to that individual. Informed dispersal led to discovery of a less dense patch than the population of origin on average. Both density and immigrant information seemed to present very similar advantages early in the evolutionary process (Fig. S5a, b and Fig. 5A). However, the benefit of informed dispersal was extremely slight (0.5%–0.02%) since the landscape was largely homogenous and most populations were very close to their carrying capacity at all times. Environmental stochasticity augmented spatial heterogeneity in patch density and led to a larger benefit during the evolution of informed dispersal (Fig. 5A; Fig. S5; see also McPeek & Holt, 1992; Travis & Dytham, 1999).

Evolution of information use: multiple sources of information

Coexistence of density and immigrant dependent dispersal occurred often in our model when both forms of information use were possible (48% of simulations for model D&I; Figs. 2C and 3). Information-dependent dispersal coefficients for both behaviors (D and I) were again significantly biased towards positive values overall in contrast to expectations from drift (Sign test for D&I model: D: 65/100 positive trials, P = 0.035; I: 82/100 positive trials, P < 0.0001). Reaction norms of density- and immigrant-dependent dispersal both show increases with density or immigrant number respectively and rise well above background levels of uninformed dispersal (Fig. 4C, D). If we contrast these reaction norms to the reaction norms that evolve when only one form of information use is possible, we see that the slope of density dependent dispersal decreases considerably (Fig. 4A vs. C) whereas the shape of the immigrant-dependent dispersal curve changes only slightly (Fig. 4B vs. D; dispersal above 98% at 3 vs. 5 immigrants respectively). Optimal levels of density-dependent dispersal therefore shift considerably when another source of information affects dispersal. In contrast, immigrant-dependent information has large effects on dispersal with the arrival of the first few immigrants and this trigger does not change much when other sources of information are available.

While both forms of dispersal evolved less often when both were present (a decrease of 32% and 17% for density and immigrant dependent dispersal respectively), coexistence remained high when in competition with a second source of information (D&I) relative to models where just one strategy was possible (D-only or I-only; Fig. 3). Joint evolution of both information use behaviors occurred even in competition with uninformed baseline dispersal (see SOM, Figs. S9, S10, S11).

Informed dispersal showed benefits at the metapopulation level when both forms of information were used together relative to using just one source of information. This benefit was most apparent when demographic stochasticity increased. Lower population carrying capacities raised the risk of extinction due to increased demographic stochasticity, and for a narrow window of carrying capacities the use of two sources of information helped reduce the risk of extinction for the metapopulation as a whole by 20%–40% relative to use of just one source of information (Fig. 5D). At slightly lower carrying capacities, when metapopulation extinction always occurred, the use of two different sources of information lead to longer persistence (200–10000 time steps or roughly 100–5000 generations; Fig. S3) of the metapopulation than if just one source of information was used. An increase in the frequency of environmental stochasticity lead to higher metapopulation extinction, and the risk of extinction was lower when one or more sources of information was available (D-only or I-only or D&I) compared to uninformed dispersal only (U-only) (Fig. 5C).

Discussion and Synthesis

Our results show that informed dispersal evolves under a broad array of contexts and that both density and indirect immigrant-dependent information sources evolve and can coexist. The frequent evolution of informed dispersal in the very conservative setup examined here (e.g. stable metapopulation) suggests that use of a variety of information sources, including indirect measures of the metapopulation landscape, could be common in nature. Indeed, direct information use in dispersal decisions is widespread (Ims & Hjermann, 2001; Matthysen, 2005; Ronce, 2007; Clobert et al., 2009; Schmidt, Dall & Van Gils, 2010) and the few empirical investigations of indirect information use that we are aware of have found evidence for it despite a broad taxonomic range. For example, common lizards modify their dispersal behavior in response to immigrants who appear to provide information about the density of their natal population (Cote & Clobert, 2007a). Likewise, our recent work in Tetrahymena ciliates shows that residents alter their dispersal rate when arriving immigrants come from populations that differ in density or social structure. In both of these empirical examples, immigrants carry more information (e.g. population density) than we included in our model. This additional information should serve to increase the fitness benefits of immigrant-dependent dispersal suggesting that we have probably underestimated the likelihood that it evolves.

For use of both information sources to evolve, there must be benefits to adjust behavior using two sources of information rather than a single source. Benefits of density-dependent dispersal are well known since movement out of high density patches should have direct fitness benefits when reproduction is density dependent (Travis, Murrell & Dytham, 1999; Matthysen, 2005; Cote, Boudsocq & Clobert, 2008). Our results demonstrate that even under very conservative conditions, immigrant dependent dispersal also presents a benefit and evolves. Likewise, coexistence of density- and immigrant-dependent dispersal even under the stable meta-population structure that we modeled suggests that these behaviors can evolve and coexist frequently even when the benefits of each behavior are low. Coexistence also implies that neither source of information carries benefits that would cause competitive exclusion of the other information source. Using two sources of information also provided additional benefits and could play an important role in metapopulation stability, especially as increased stochasticity creates larger inequalities in population densities. The benefits of using multiple information sources (decreased extinction risk) that we measured occurred for a small range of meta-population conditions (medium levels of stochasticity), but they suggest an important advantage to informed dispersal under less stable conditions of most real meta-populations. While the benefits we measured in our model were small in the relatively homogenous landscape we constructed, conditions that more realistically imitate empirical landscapes should confer much larger benefits to this behavior.

Joint evolution of density and immigrant dependent dispersal would be prevented if information content of density and immigrant number were not sufficiently different or if one information source was superior to the other (Enfjäll & Leimar, 2009; Hovestadt, Kubisch & Poethke, 2010; Schmidt, Dall & Van Gils, 2010; Bocedi, Heinonen & Travis, 2012). Immigrant arrival might be related to the overall density of the metapopulation since populations that have more individuals will generate more dispersers, and therefore immigrants, even through a fixed baseline dispersal rate. This estimate of the overall metapopulation density contrasts to density-dependent measures of the local population alone. Competitive exclusion might be expected since immigrant number should be more decoupled with local fitness in any single patch and thus dispersal should carry a higher variance in benefits relative to direct information on local density. In simulations where we introduced a difference in the cost of using each form of information (Figs. S6, S7), the most costly form of information did not evolve – although this cost is not directly linked to the quality of information. More frequently, we found coexistence of information use through both density and immigrant information. This suggests that each source of information is not fully redundant and that one source of information is not necessarily superior to the other. This equivalency of information can serve as an advantage under some contexts (e.g. when stochasticity is high; Figs. S5 and S3) and would be especially useful where the costs of information use from one source might constrain dispersal below an optimal level (Bocedi, Heinonen & Travis, 2012). Likewise, if immigrants also carry additional information about their populations (Cote & Clobert, 2007a) or help orient dispersers towards certain populations, then we could expect the benefits of indirect information use to be even more advantageous.

The potential prevalence of informed dispersal has a number of important implications for both fundamental and applied ecology. In basic ecological research, the use of information has recently been explored in terms of density dependent dispersal, and this simple behavior greatly effects how movement influences population persistence (Ims & Hjermann, 2001; Cadet et al., 2003; Matthysen, 2005). Earlier models of “informed” dispersal – such as “ideal free distribution” models – generally assumed perfect knowledge of the landscape (Abrahams, 1986; Gray & Kennedy, 1994; Holt & Barfield, 2001) which presumably was acquired through prospecting that carried low costs. Low cost prospecting might work when patches are close (e.g. foraging patches), but is less realistic when habitat patches are more distant. The use of indirect information, such as the arrival of immigrants, could provide another mechanism by which the ideal free distribution is achieved (Baguette, Clobert & Schtickzelle, 2010). If immigrant arrival is linked to overall metapopulation density and if immigrants carry additional information about the quality of those habitats as suggested in empirical examples (Cote & Clobert, 2007a), then we might approach an ideal free distribution through use of indirect information transfer across the landscape. Deviation from ideal free models might then in part reflect the quality or reliability of that indirect information transfer (see also Abrahams, 1986; Gray & Kennedy, 1994; Chaine & Clobert, 2012). Most likely, individuals use a number of sources of information on local conditions, direct prospecting of nearby patches, and indirect measures of the landscape such as immigrant-borne information (Clobert et al., 2009). If this form of information use is prevalent, then we must shift our view of dispersal from largely random movement among populations to much more targeted and informed movement patterns that approach ideal-free expectations.

Connectivity and dispersal are crucial aspects of population persistence, yet studies of dispersal and metapopulation dynamics usually ignore the important role that information transfer across the landscape might play in guiding subsequent dispersal decisions. Applied management or conservation efforts to increase connectivity or gene flow might be greatly hampered if we do not also introduce the indirect cues that influence dispersal. Indeed, the highly variable success of artificial corridors (Gilbert-Norton et al., 2010) could in part be caused by the lack of indirect information since immigrants will be rare when a new corridor is first constructed (see also Le Galliard, Ferriere & Clobert, 2003). More generally, conservation efforts could be greatly aided by modifying natural dispersal through the manipulation of information that is accessible to residents rather than by costly alterations of the landscape between habitat patches (Chaine & Clobert, 2012). As we show here, access to multiple sources of information may better mitigate extinction risk in highly stochastic environments compared to situations where little information exists. Broader inclusion of how information is used in dispersal should provide us with new tools for conservation and fundamentally modify our approach to conservation ecology and the management of populations in peril.

Our findings also have important implications for dispersal theory and the incorporation of information use into this field. We found the evolution of both forms of informed dispersal despite potentially large differences in the quality of information gleaned from each source. Whereas local density directly affects fitness, immigrant arrival at best gives some indication of surrounding population sizes when density dependent dispersal exists and at worst simply provides evidence that other populations exist. Coexistence of the two sources of information suggests that the quality of information may be somewhat less important than the presence of that information. In support of this notion, models of indirect information use based on immigrant presence rather than immigrant number show very similar results (Fig. S12). This result is empirically supported by the fact that dispersal in the common lizard was found to be sensitive to the presence and not to the quantity of immigrants (Cote & Clobert, 2007a). Similarly, recent models of density dependent information use suggest that the precision of information provides diminishing returns and high quality information is not optimal when it also incurs elevated costs associated with gathering additional precision (Bocedi, Heinonen & Travis, 2012). Both of these investigations adopt very simple dispersal contexts and yet both show that information use in dispersal evolves quite readily and should be common in nature. More generally, the passive information transfer across the landscape that evolves in our models could be an important first evolutionary step that allows more active information transfer and communication to evolve both within populations and across landscapes.

Supplemental Information